[eschewthecashew]

What exactly does it mean for one molecular orbital to push another up or down in energy?

[Corribus]

It means the molecular orbitals are mixing character, which can happen if they have mutually appropriate symmetry. The degree of mixing depends on spatial overlap as well as relative energy.

A classical analogy is if you bring two bar magnets close together.  Each magnet in isolation has a certain amount of reference energy.  Now when you bring them together, the new potential energy will depend on their mutual orientation. First, suppose you bring them together in perpendicular orientation, with either head of one approaching the middle of the other, to form a "T" shape. Here the symmetries of the two are not compatible, and what you get is no interaction at all. They neither repel or attract. The potential energy of the system doesn't change.

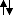
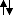
Now, suppose you bring them in parallel to each other, like this: or  (the arrow indicates the direction of the magnetic moment). There are two possible ways you can bring them together, as indicated. One is that they are head to head (parallel,  ) and the other is that they are head to tail (antiparallel,  ). In both cases the symmetry is appropriate for interaction, but the type of interaction is different. In a head to tail orientation, there is an attractive force, which lowers the potential energy of the system relative to the potential energy of two isolated magnets (you'd have to supply work to separate them), and the degree of energy lowering depends on the starting strength of the magnets and how close you bring them together.  In a head to head orientation, the magnets repel each other and the potential energy is raised compared to the potential energy of two isolated magnets. The degree of repulsion when antiparallel is ~ equal to the degree of attraction in the opposite, parallel case.

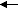
A final case is if you bring the two magnets together so that they are laying in a single line, like this:  or  . Again the symmetry is appropriate for mixing, and we have the same possibilities as before: a head to head and a head to tail. And as before a head to tail results in a lowering of the potential energy (they stick together) and a raising of the potential energy (they force apart), respectively. 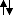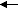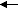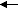The only real difference between  / and  / is the magnitude of interaction: the potential energy changes of / are actually greater (twice larger, in the point dipole approximation), because the parts of similar polarity are farther from each other (or twice closer, for the unfavorable  interaction) than if they are / .

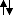
This may seem rather abstract but it's actually fairly relevant to orbital interaction, which is governed in large part by the interaction of the magnetic fields created by moving electrons. In fact, p-orbitals interact in a very similar way to classical magnetic dipoles, the difference being that energies and other observables are quantized. Energies of interaction can take on only certain values and this restricts where electrons in those orbitals can be found in space. Two p-orbitals interacting in a  orientation would be a sigma bond interaction between two adjacent p-orbitals and the  would be a sigma antibonding interaction between two adjacent p-orbitals, with corresponding lowering and raising of the interaction (bond/antibond) energy compared to isolated p-orbitals. You probably can guess that the  and  interactions correspond to pi-bonding and pi-antibonding interactions, respectively. And yes, as the simple classical point dipole interaction suggests, pi-bonds are weaker than sigma bonds because the  -type interactions are generally weaker than  -type interactions. In QM formulation we speak of Coulomb, exchange and overlap integrals, but it is effectively the same thing with the same general result.  Finally, if you tried to bring together two p-orbitals in a head to waist orientation  , there is no bonding interaction at all because of the way the fields cancel each other out. The symmetry isn't right.

Also note here that the dependence of the "pushing" effect, as you call it, on the relative starting energies of the isolated orbitals is analogous to combining classical magnets of different strengths. If you take a really strong bar magnet and put it next to a really little bar magnet, the same types of interactions will occur, but the relative interaction energy will be smaller. (A weak magnet isn't going to perturb the energy of the strong magnetic as much as another strong magnet would). This is why 1s orbitals don't interact much with 4s orbitals, for example, even though they have the appropriate symmetry to do so (proximity of the orbitals is also a factor here as well, to be fair).

Interactions between d-orbitals and s-orbitals work essentially the same way as everything described above, but now your symmetry combinations are different and cannot be compared so neatly to the classical analogy of a bar magnet.

Note that this analogy also applies to other molecular orbital interactions such a J- and H-aggregates, excitons in conducting polymers, and so on.

[eschewthecashew]

Wonderful explanation, especially in context of magnetism. Thank you. 


Three orbital interactions are a little bit harder.  I'm still unsure about what dictates the type of interaction (constructive or destructive) the atomic orbitals will have in this scenario.  Also, I'm confused about atomic orbital interactions vs molecular orbital interactions. 

For example, molecular orbital theory can be used to explain the bending of ammonia.  I understand that the bending the molecule changes the symmetry such that the lone pair mostly distributed on nitrogen's pz orbital will have the appropriate symmetry to interact with other orbitals that will lower its energy.  My argument is that the lowering in energy is due to mixing/interaction of the nitrogen 2s atomic orbital with the nitrogen 2pz orbital.  My professor says it is due to interaction of molecular orbitals where the A1 antibonding orbital "pushes" Pz down in energy.  I don't get this...

[Corribus]

Ok, first you have to realize that molecular orbitals are a human theoretical construct created to explain observations. They also aren't the only game in town. There are other bonding theories that can be used. Sometimes MO theory works best. Other theories may function better in other situations. Which is to say, there is more than one way to explain what's going on in any situation, and often two explanations can both be correct because fundamentally they are exactly the same, even if they don't seem so on the surface.

Atomic orbitals were devised to explain the quantized electronic interactions with a single atomic nucleus. Important to understand that with the exception of hydrogenic atoms, AOs are based on approximations that make the problems possible to be solved. Such as assuming that electrons in the same atom do not interact with each other, for instance, and interact with the nucleus only through a static, effective charge field.

With the early successes of atomic structure and quantum mechanics behind them, quantum theorists were forced to try to explain how electrons in one nucleus interact with nearby nuclei and their surrounding electrons. An early approximation was made that the quantized energies and spatial arrangements of electrons in poly-nuclear systems where combinations of the respective atomic orbitals. This was the beginning of molecular orbital theory, and particular LCAO-MO (linear combination of atomic orbital-molecular orbital) theory. More, it was really the beginning of quantum chemistry, because prior to this point, quantum theory really was only relevant to atomic structure and couldn't explain much in terms of the way atoms bonded together. What a victory!

Now, you can build molecular orbitals as combinations of atomic orbitals, as described in my earlier post. You can also build molecular orbitals in terms of simpler molecular orbitals. At that point it's all just math. You can also new molecular orbitals by considering how molecular orbitals interact with other nearby atomic orbitals. This is often an important consideration in transition metal chemistry, where metal d-orbitals interact with the molecular orbitals in ligands.

Anyway, it always just boils down to interacting magnetic and electric fields. The orbitals, remember, are just a convenient shorthand way to visualize how electrons move about in space based on their way they interact with the field lines created by other nearby charged particles. Of course the rules that govern these motions are quantum mechanical rather than classical mechanical, so some of the results are surprising.

Three orbital combinations are thus no different really from two orbital combinations. When you have two interacting orbitals, you have two ways they can interact, as discussed before. If you have three interacting atomic orbitals, you have three ways they can interact: you form three molecular orbitals. And as before, the energies and shapes of these orbitals will be a complex function of their relative orientation, energy, and proximity to one another.

In the case of ammonia, your professor's approach is to consider the way that a molecular orbital involving four atoms interact with a "nonbonding" atomic orbital on nitrogen. Assuming the symmetries are appropriate, there is nothing wrong with this kind of approach - and if they do interact, they will form two effective new orbitals, one with a slightly lower energy and one with a slightly higher energy. Alternatively, you can just start from scratch and consider the way all the atomic orbitals interact. This latter approach is similar to the way atomic orbital hybridization is usually formulated. Both approaches have their advantages and weaknesses.  Just remember that neither approach is, strictly speaking, "the true explanation". These are just mathematical approaches to explain The Fact (in this case, ammonia is bent).

The reason your professor's approach is often used to explain things like the bending of ammonia is that you don't have to start from scratch (i.e., at the atomic orbital stage) to understand structural changes as you swap out atoms - such as going form BH3 to NH3. I'm not sure if you are familiar with Walsh diagrams or not, but let's say you wanted to know why BH3 is planar and NH3 is not. Well, you could in each case start with all the atomic orbitals, calculate how they interact with each other, and predict the resulting molecular structure. That's a lot of work for one molecule, let alone two (or fifty, if you want to know how CH3 looks, and SiH3, and so on). Alternatively, you can realize that in most cases the atomic orbitals involved will be identical, and it's just the number of electrons that fill them up that is mostly changing. So you can plot out the molecular orbital energies (AO-AO and AO-MO interactions) as a function of various structural variations (such as pulling the central atom away form the plane) to predict what the favored structural will be for a given molecular formula.

Probably that will be hard to follow if you don't know what a Walsh diagram is. I guess the point is that if you want to know why NH3 is not planar, it's easiest to start with the base set of molecular orbitals for a four atom system and then try to understand how they will interact with other electrons (and their orbitals) that are relevant to the specific system you're interested in. The standout feature of NH3 is the extra pair or electrons in nitrogen. So you take your standard AB3 molecular orbitals and then look at how those MO's will interact with the extra pair of electrons on nitrogen. You soon see (by introspection or calculation) that one of the MO's is appropriate symmetry to interact with that nitrogen lone pair atomic orbital. Any such interaction will "push down" one orbital and "raise up" the other, at least as far as energy is concerned, as discussed earlier. In addition to energy changes, though, another ramification of orbital interaction is mixing of character. What this means is that if orbital A and orbital B interact, you don't just get a lower energy orbital A and a higher energy orbital B. What you get are two new orbitals that have a mixture of the structural characteristics of A and B (A + B and A - B), with the relative % character depending on the relative starting energies. So if you have a planar nitrogen molecular orbital mixing with an AO oriented perpendicular to the plane, what you're going to get is a new molecular orbital that's oriented partially out of the plane - that is, nitrogen will be bent out of the plane because it has some of that pz atomic orbital character in it.

I hope all of that rambling made a little bit of sense.